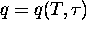
- ...
- In order to simplify the notation
we do not write explicitely the dependence of the
concentrations on
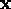, but actually the concentrations are functions of both
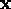, T and 
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...constant
- E.J. Hinch, Perturbation Methods (Cambridge University Press, Mexico, 1992), p. 116.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...stable
- Given
that (20) defines a continuum of fixed points, they are all
``marginally'' stable, in the sense that the linearization of the dynamical system
around any of them has a zero eigenvalue. However, the system also has N constants of
motion so that only one fixed point belongs to each level set of these N constants.
We discuss this in detail in Sec. III.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...
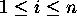
- Notice
that the right-hand-sides of Eqs. (25)-(26) correspond to a
linearization of the system (10)-(11) around a fixed point given
by (20). As mentioned before, this linearized operator has eigenvalues equal to zero.
Requiring that
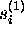for all i is equivalent to saying that the solutions
, 
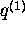do not have any component along the directions associated with these
zero eigenvalues.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...in
- J.E. Pearson, and W. Bruno, Chaos 2, 513 (1992);
S. P. Dawson, A. Lawniczak, and R. Kapral, J. Chem. Phys. 100,
5211 (1994)
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...model
- E. E. Selkov, European
J. Biochem. 4, (1968),p. 79-86.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...in
- L. A. Segel and M. Slemrod, SIAM Rev. 3, (1989), p.466-477.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...in
- E.C. Edblom, M. Orban, and I.R. Epstein,
J. Am. Chem. Soc. 108, 2826 (1986)
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...in
- K.J. Lee, W.D. McCormick, Q. Ouyang, and H.L.
Swinney,
Science 261, 192 (1993);
K.J. Lee, W.D. McCormick, J.E. Pearson, and H.L.
Swinney, Nature 369, 215 (1994); K.J. Lee, and H.L.
Swinney, Phys. Rev. E 51, 1899 (1995)
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...in
- V. Gáspár, and K. Showalter, J. Phys. Chem. 94, 4973 (1990)
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.